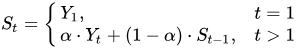I am trying to implement Exponential Moving Average calculation on a DataFrame. The formula is
An additional complication is that my table is grouped and there is a unique bin number per group. This is what I tried
import numpy as np
import numpy.random as rand
n = 5
groups = np.array(['one', 'two', 'three'])
data = pd.DataFrame({
'price': rand.random(3 * n) * 10,
'group': np.repeat(groups, n),
'bin': np.tile(np.arange(n),3)}, index=np.arange(3 * n))
print(data)
price group bin
0 1.601310 one 0
1 3.190662 one 1
2 4.419421 one 2
3 3.817510 one 3
4 2.440774 one 4
5 6.832265 two 0
6 5.636502 two 1
7 4.630515 two 2
8 5.856423 two 3
9 0.916452 two 4
10 4.247134 three 0
11 7.146746 three 1
12 8.049161 three 2
13 7.852168 three 3
14 0.246720 three 4
This is how I am trying to implement the EMA calculation;
data['EMA'] = np.zeros(len(data.index))
data.loc[data['bin'] == 0, 'EMA'] = data.loc[data['bin'] == 0, 'price']
a = 2 / (n + 1)
for _, group in data.groupby('group'):
for index, row in group.iloc[1:].iterrows():
prev = group[group['bin'] == row['bin'] - 1].iloc[0]
row['EMA'] = a * row['price'] + (1 - a) * prev['EMA'] # nope
data.loc[index, 'EMA'] = a * row['price'] + (1 - a) * prev['EMA'] # nope
Unfortunately neither of these last lines update the values in the group. On the second iteration, the value of prev['EMA'] is still 0. What is the correct way to assign the values back to the table such that it is updated dynamically? Do I need to write out to a temporary array and write it back afterwards?
Additionally, I could not think of an elegant way to do this using assign or transform. If someone can solve that it might be a very good alternative.
Response
Thank you @DaFanat for your help. Unfortunately your code doesn't work. I tried the following
data.loc[:, 'EMA2'] = map(lambda x, y: x if pd.isnull(y) else x*a + (1-a) * y,
data['price'], data.groupby('group')['price'].shift(1))
But I get an error TypeError: object of type 'map' has no len(). I tried changing it to this
data['EMA2'] = list(map(lambda x, y: x if pd.isnull(y) else x*a + (1-a) * y,
data['price'], data.groupby('group')['price'].shift(1)))
And I do indeed get some results, but they do not look correct;
price group bin EMA EMA2
0 5.407722 one 0 5.407722 5.407722
1 0.495734 one 1 3.770393 3.770393
2 7.911491 one 2 5.150759 2.967653
3 1.085836 one 3 3.795785 5.636272
4 7.326432 one 4 4.972667 3.166035
I am inclined to believe my implementation, since the price goes up from 0.5 to 7.9, how can the moving average go down? I think the indices are getting lost and it is putting the values against the wrong cells. How do I retain the indices when performing this calculation?
Solution
Thanks @DaFanat, you took me a long way. I finally figured out how to do it with a slight modification of your original method;
data['EMA2'] = data.groupby('group') \
.apply(lambda x: x['price'] * a + x['EMA'].shift(1) * (1-a)) \
.reset_index(level=0, drop=True)
data.loc[data['bin'] == 0, 'EMA2'] = data.loc[data['bin'] == 0, 'price']
print(data)
price group bin EMA EMA2
0 3.556171 one 0 3.556171 3.556171
1 5.637241 one 1 4.249861 4.249861
2 3.278771 one 2 3.926164 3.926164
3 7.343718 one 3 5.065349 5.065349
4 6.128884 one 4 5.419861 5.419861
Not using the list(map()) ensures that the result is a DataFrame with indices retained, so it knows where to insert the individual rows.