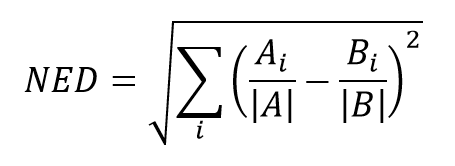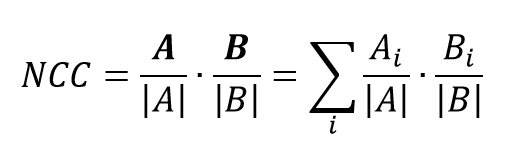3
$\begingroup$

Normalized Euclidean Distance and Normalized Cross - Correlation can both be used as a metric of distance between vectors.

What is the difference between these metrics? It seems to me that they are the same, although I have not seen this explicitly stated in any textbook or literature.

thank you.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

These two metrics are not the same.

The normalized Euclidean distance is the distance between two normalized vectors that have been normalized to length one. If the vectors are identical then the distance is 0, if the vectors point in opposite directions the distance is 2, and if the vectors are orthogonal (perpendicular) the distance is sqrt(2). It is a positive definite scalar value between 0 and 2.

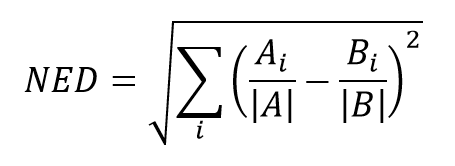
NED equation

The normalized cross-correlation is the dot product between the two normalized vectors. If the vectors are identical, then the correlation is 1, if the vectors point in opposite directions the correlation is -1, and if the vectors are orthogonal (perpendicular) the correlation is 0. It is a scalar value between -1 and 1.

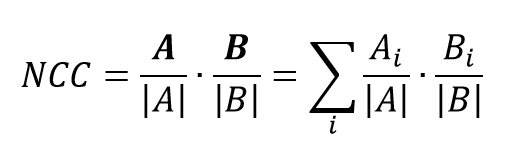
NCC equation

This all comes with the understanding that in time-series analysis the cross-correlation is a measure of similarity of two series as a function of the lag of one relative to the other.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Nice, good explanation. $\endgroup$
    – makansij
    Commented Jul 24, 2015 at 22:09
  • $\begingroup$ The one part about this that is unclear to me, is - how does this account for the variance in the denominator? It was my understanding that NCC is normalized by the variance of the function. Is that true? I'm not seeing this in your explanation. $\endgroup$
    – makansij
    Commented Jul 31, 2015 at 9:33
  • $\begingroup$ The distinction between standardizing the cohort and normalizing the individual vectors is confusing when NCC is put in functional form. I'll try to edit my answer over the weekend to hash this out. $\endgroup$
    – AN6U5
    Commented Jul 31, 2015 at 15:54
  • $\begingroup$ Have you figured this out? Thanks. $\endgroup$
    – makansij
    Commented Aug 4, 2015 at 19:57
  • $\begingroup$ I think I get it @an6u5. $\endgroup$
    – makansij
    Commented Aug 5, 2015 at 17:30

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.