I am trying to find out if stock market movements, on average and in extreme conditions, affect gold prices. I am following the regression model proposed by Baur and McDermott (2010) which is given as:
$R_{asset,t}=a+b_tR_{stock,t}+\epsilon_t$
$b_t=c_0+c_1D(R_{stock}q_{10})+c_2D(R_{stock}q_{5})+c_3D(R_{stock}q_{1})$
$h_t=\omega+\alpha\epsilon_{t-1}^2+\beta h_{t-1}$
All models are estimated simultaneously with maximum likelihood methods as mentioned in their published paper which I do not know how to apply.
Below is what I have done:
reg <- read.csv(file = "MVreturnsqreg.csv")
The csv file contains time series of gold, S&P500 10 Quantile, S&P500 5 Quantile, S&P500 1 Quantile.
I used the following regression command in R which I am not sure if it is the correct way to do and whether I can use OLS-regression with time series data. (If not, what is the right type of regression?)
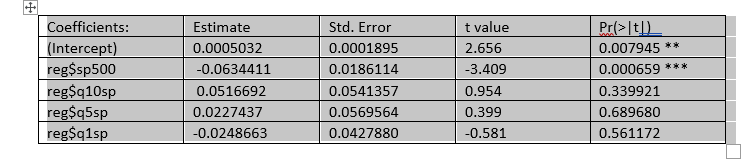
goldregression= lm (reg\\\$gold ~ reg\\\$sp500 + reg\\\$q10sp + reg\\\$q5sp + reg\\\$q1sp)
Below is the output of the regression model, but the estimates at all quantiles are not significant.
Also, I do not know how to take heteroskedasticity into consideration? I know GARCH (1,1) can take care of that, but how do you estimate it with other models (1,2) at the same time? How can I Incorporate the above OLS-Regression into a GARCH-model in order to receive fitted coefficients? Or how do you fit a GARCH (1,1) model into the regression model? I do not know which way it works.
If I use the "rugarch" package to model a GARCH(1,1), I would only get the parameters regarding the volatility equation $(\mu, \omega, \alpha, \beta)$, but not the coefficients of my independent variables. If I have to use the GARCH method, where do I find the estimations for my independent variables after using the GARCH?
Any suggestions?